Objective

To look at the Least Common Multiple, LCM, and Greatest Common Factor, GCF, from "hands-on" to using sets.

Audience

This activity is intended for teachers. The activity is designed to make connections between the use of manipulatives and the more formal use of sets in number theory. Parents and students are welcomed!

Materials Needed - Virtual Manipulative Integer Rods!

Instructions
You may want to print this page for documentation.
• Open the virtual Integer Rods.
• Click on the rod on the left to select and drag a rod to the work area.
• Click on the icons on the top of the screen to be able to select, rotate, lasso rods, exchange rods, and choose options.
• Click on the broom to clean up! You can also drag rods to the trash!

Developed by Jacobo Bulaevsky.

LCM from Different Points of View

1. The rods shown below show two trains, one built with 4's the other with 6's. Notice that there are 3 groups of 4 and 2 groups of 6 before the trains are the same length. You can say that 12 is a common multiple of 4 and 6!
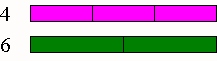   Use the rods and try to find the next time the 4-train will be the same length as the 6-train. This will be another common multiple. Record the length in the table. What is the next time the lengths match up, the next multiple? ...and the next multiple? Fill in the table. (Keep the rods together for more questions later!)

Train size
Multiples of 4
Multiples of 6

To find the Least Common Multiple, you need to:
• Find the common multiples of the numbers.
• Find the smallest number. This is the "least."


Look back at your table, write the least common multiple of 4 and 6, LCM(4,6).


2. Using Sets
   Notice that you can see, using the rods, that 4, 8, and 12 are multiples of 4 and 6 and 12 are multiples of 6! Now, use your trains made in part 1 to list the multiples of 4 and 6.

Multiples of 4 = { , , ; , , ...}

Multiples of 6 = { , , ; , , ...}


Next, list the numbers that are common to these sets. Find the intersection of the sets.


Common Multiples of 4 and 6 = { , , ; , , ...}

Next, pick the smallest number in the set of common multiples. Write the Least Common Multiple of 4 and 6 = LCM(4,6)=

Problems

3. If you are adding or subtraction fractions with unlike denominators, you can use a common multiple of the denominators to rename the fractions. Add these fractions using the information found above.



4. Find the LCM(12, 15) using rods. Print out or draw your answer on the grid given to find the LCM (12, 15).


Multiples of 12 = { , , ; , , ...}
Multiples of 15 = { , , ; , , ...}

Common Multiples of 12 and 15 = { , , ; , , ...}

Least Common Multiple of 12 and 15 = LCM(12,15)=

5. Use a common multiple to subtract these fractions. Show all steps!



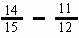
Applications



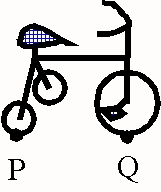
6. The front wheel of a tricycle has a circumference of 54 inches and the back wheels have a circumference of 36 inches. If points P and Q are both touching the sidewalk when Jose starts to ride, when will P and Q first touch the sidewalk at the same time again? Which tire would wear out first? Explain.









7. Two of your children ride 2 wheel bikes to school. Karen has a bike with wheel size 18 inches. Maria has a bike with wheel size of 26 inches. Karen was very tired and could not keep up with Maria on their ride to school. Explain to Karen why she had a different experience than Maria.









Finding the Greatest Common Factor of Two Numbers
8. The rods shown below show the factors of 6 and 8. Look at the rods and write down the factors of 6 and the factors of 8.



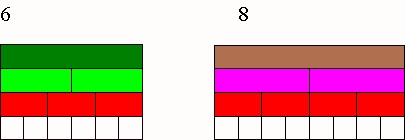
Factors of 6 = { , , ; , , ...}

Factors of 8 = { , , ; , , ...}

Common Factors of 6 and 8 = { , , ; , , ...}

To find the Greatest Common Factor, you need to:
• find the factors for each number.
• find the common factors.
• find the largest of these common factors. This is the "greatest."
• look back at your table, what is the greatest common factor of 6 and 8? Write down the GCF(6,8).

Problems

9. Use the GCF(6, 8) to simplify the following fraction. Show all steps!



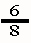
10. There are three 4^th Grades, A, B, and C, at the Local Elementary School. Room 4-A contains 12 children, Room 4-B contains 18, and Room 4-C contains 24 children. The 4^th graders will get together to play a game in the middle of the day. The teachers want the children to be on teams with their own classmates but all teams need to be of equal size. Can you find the size of the teams so that everyone plays and each team contains children from the same room?









11. Use the rods to find the GCF(6,9). Draw your work on the grid below.



Factors of 6 = { , , ; , , ...}

Factors of 9 = { , , ; , , ...}

Common Factors of 6 and 9 = { , , ; , , ...}

Write the Greatest Common Factor of 6 and 9 = GCF(6,9)

Use the GCF(6, 9) to simplify the following fraction. Show all steps!


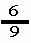
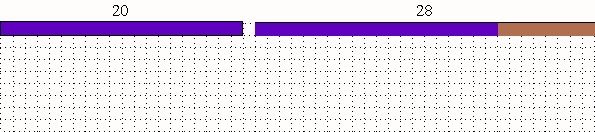
12. Use the rods to find the GCF(20, 28). Draw your work on the graph below.



Factors of 20 = { , , ; , , ...}

Factors of 28 = { , , ; , , ...}

Common Factors of 20 and 28 = { , , ; , , ...}

Write the Greatest Common Factor of 20 and 28 = GCF(20,28) =

13. Use the GCF(20, 28) to simplify the following fraction. Show all steps!

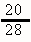
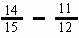
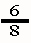
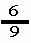
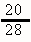
Copyright 1998 by Margo Lynn Mankus